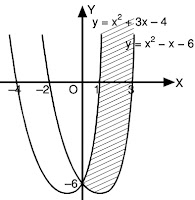
y ≤ x2 + 3x – 4 y ≥ x2 – x – 6
Gambarlah grafik himpunan penyelesaiannya!
y ≤ x2 + 3x – 4
y ≥ x2 – x – 6
Jawab:
y ≤ x2 + 3x – 4
Titik potong sumbu-x (y = 0)
x2 + 3x – 4= 0
(x + 4)(x – 1) = 0
x = -4 atau x = 1
(-4, 0) (1, 0)
Titik potong sumbu-y (x = 0):
y = 02 + 3(0) – 4 = -4
(0, -4)
Sumbu simetri:
x = -b/2a = - 3/2. 1 = 3/2
yp = (3/2)2 + 3(3/2) – 4 = 2,25 – 4,5 – 4 = -6,25
(3/2, -6,25)
y ≥ x2 – x – 6
Titik potong sumbu-x (y = 0)
x2 – x – 6 = 0
(x + 2)(x – 3) = 0
x = -2 atau x = 3
(-2, 0) (3, 0)
Titik potong sumbu-y (x = 0):
y = 02 – 0 – 6 = -6
(0, -6)
Sumbu simetri:
x = -b/2a = - -1/2. 1 = ½
yp = (½)2 – ½ – 6 = 0,25 – 0,5 – 6 = -6,25
(½, -6,25)
Sehingga diperoleh grafiknya:
----------------#----------------
Semoga Bermanfaat
Jangan lupa komentar & sarannya
Email: nanangnurulhidayat@gmail.com
Post a Comment for "y ≤ x2 + 3x – 4 y ≥ x2 – x – 6"